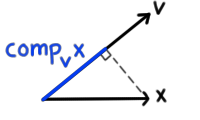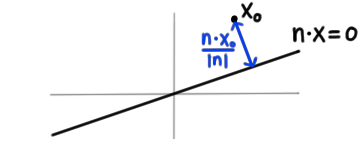Consider the point $\bfx_0 = (x_0, y_0)$ and the line given by $\bfn \cdot \bfx = 0$, where $\bfn = (a, b)$. Show that the minimum distance from $\bfx_0$ to the line $\bfn\cdot\bfx=0$ is $\frac{\left |\bfn\cdot \bfx_0 \right|}{\left| \bfn \right| }$.